Usuário:JMagalhães/traduções11

Diagramas de Coxeter–Dynkin diagrams for the fundamental finite Coxeter groups

Coxeter–Dynkin diagrams for the fundamental affine Coxeter groups

Em geometria, um diagrama de Coxeter-Dynkin é um grafo com extremidades numeradas (designadas por ramos), que representa as relações espaciais entre uma série de espelhos (ou hiperplanos reflectores). Define uma construção caleidoscópica: cada "nó" no grafo representa um espelho

In geometry, a Coxeter–Dynkin diagram (or Coxeter diagram, Coxeter graph) is a graph with numerically labeled edges (called branches) representing the spatial relations between a collection of mirrors (or reflecting hyperplanes). It describes a kaleidoscopic construction: each graph "node" represents a mirror (domain facet) and the label attached to a branch encodes the dihedral angle order between two mirrors (on a domain ridge). An unlabeled branch implicitly represents order-3.

Each diagram represents a Coxeter group, and Coxeter groups are classified by their associated diagrams.

Dynkin diagrams are closely related objects, which differ from Coxeter diagrams in two respects: firstly, branches labeled "4" or greater are directed, while Coxeter diagrams are undirected; secondly, Dynkin diagrams must satisfy an additional (crystallographic) restriction, namely that the only allowed branch labels are 2, 3, 4, and 6. See Dynkin diagrams for details. Dynkin diagrams correspond to and are used to classify root systems and therefore semisimple Lie algebras.

Description

Branches of a Coxeter–Dynkin diagram are labeled with a rational number p, representing a dihedral angle of 180°/p. When p = 2 the angle is 90° and the mirrors have no interaction, so the branch can be omitted from the diagram. If a branch is unlabeled, it is assumed to have p = 3, representing an angle of 60°. Two parallel mirrors have a branch marked with "∞". In principle, n mirrors can be represented by a complete graph in which all n(n − 1) / 2 branches are drawn. In practice, nearly all interesting configurations of mirrors include a number of right angles, so the corresponding branches are omitted.

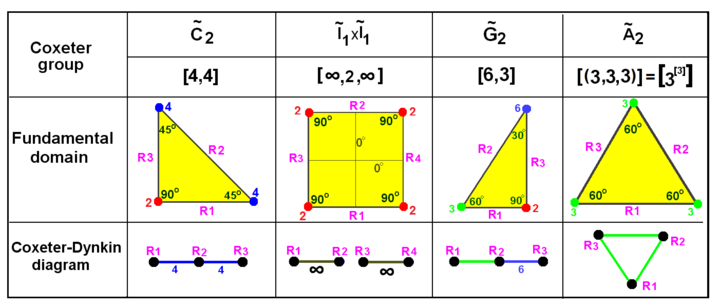
Geometric visualizations

The Coxeter–Dynkin diagram can be seen as a graphic description of the fundamental domain of mirrors. A mirror represents a hyperplane within a given dimensional spherical or Euclidean or hyperbolic space. (In 2D spaces, a mirror is a line, and in 3D a mirror is a plane).

These visualizations show the fundamental domains for 2D and 3D Euclidean groups, and 2D spherical groups. For each the Coxeter diagram can be deduced by identifying the hyperplane mirrors and labelling their connectivity, ignoring 90-degree dihedral angles (order 2).

Coxeter groups in the plane with equivalent diagrams. Domain mirrors are labeled as branch m1, m2, etc. Vertices are colored by their reflection order. The prismatic group ${\tilde {I}}_{1}$x${\tilde {I}}_{1}$ is shown as a doubling of the ${\tilde {C}}_{2}$, but can also be created as rectangular domains from doubling the ${\tilde {G}}_{2}$ triangles. The ${\tilde {A}}_{2}$ is a doubling of the ${\tilde {G}}_{2}$ triangle.

Coxeter groups in 3-space with diagrams. Mirrors (triangle faces) are labeled by opposite vertex 0..3. Branches are colored by their reflection order. ${\tilde {C}}_{3}$ fills 1/48 of the cube. ${\tilde {B}}_{3}$ fills 1/24 of the cube. ${\tilde {A}}_{3}$ fills 1/12 of the cube.

Coxeter groups in the sphere with equivalent diagrams. One fundamental domain is outlined in yellow. Domain vertices (and graph branches) are colored by their reflection order.

Application with uniform polytopes

Coxeter–Dynkin diagrams can explicitly enumerate nearly all classes of uniform polytope and uniform tessellations. Every uniform polytope with pure reflective symmetry (all but a few special cases have pure reflectional symmetry) can be represented by a Coxeter–Dynkin diagram with permutations of markups. Each uniform polytope can be generated using such mirrors and a single generator point: mirror images create new points as reflections, then polytope edges can be defined between points and a mirror image point. Faces can be constructed by cycles of edges created, etc. To specify the generating vertex, one or more nodes are marked with rings, meaning that the vertex is not on the mirror(s) represented by the ringed node(s). (If two or more mirrors are marked, the vertex is equidistant from them.) A mirror is active (creates reflections) only with respect to points not on it. A diagram needs at least one active node to represent a polytope.

All regular polytopes, represented by Schläfli symbol symbol {p, q, r, ...}, can have their fundamental domains represented by a set of n mirrors with a related Coxeter–Dynkin diagram of a line of nodes and branches labeled by p, q, r, ..., with the first node ringed.

Uniform polytopes with one ring correspond to generator points at the corners of the fundamental domain simplex. Two rings correspond to the edges of simplex and have a degree of freedom, with only the midpoint as the uniform solution for equal edge lengths. In general k-rings generators are on k-faces of the simplex, and if all the nodes are ringed, the generator point is in the interior of the simplex.

A secondary markup conveys a special case nonreflectional symmetry uniform polytopes. These cases exist as alternations of reflective symmetry polytopes. This markup removes the central dot of a ringed node, called a hole (circles with nodes removed), to imply alternate nodes deleted. The resulting polytope will have a subsymmetry of the original Coxeter group. If all the nodes are holes, the figure is considered a snub.

• A single node represents a single mirror. This is called group A₁. If ringed this creates a line segment perpendicular to the mirror, represented as {}.
• Two unattached nodes represent two perpendicular mirrors. If both nodes are ringed, a rectangle can be created, or a square if the point is at equal distance from both mirrors.
• Two nodes attached by an order-n branch can create an n-gon if the point is on one mirror, and a 2n-gon if the point is off both mirrors. This forms the I₁(n) group.
• Two parallel mirrors can represent an infinite polygon I₁(∞) group, also called Ĩ₁.
• Three mirrors in a triangle form images seen in a traditional kaleidoscope and can be represented by three nodes connected in a triangle. Repeating examples will have branches labeled as (3 3 3), (2 4 4), (2 3 6), although the last two can be drawn as a line (with the 2 branches ignored). These will generate uniform tilings.
• Three mirrors can generate uniform polyhedra; including rational numbers gives the set of Schwarz triangles.
• Three mirrors with one perpendicular to the other two can form the uniform prisms.

Example BC3 polytopes

For example, the BC₃ Coxeter group has a diagram: . This is also called octahedral symmetry.

There are 7 convex uniform polyhedra that can be constructed from this symmetry group and 3 from its alternation subsymmetries, each with a uniquely marked up Coxeter–Dynkin diagram. The Wythoff symbol represents a special case of the Coxeter diagram for rank 3 graphs, with all 3 branch orders named, rather than suppressing the order 2 branches. The Wythoff symbol is able to handle the snub form, but not general alternations without all nodes ringed.

Name	Cube	Truncated cube	Cuboctahedron	Truncated octahedron	Octahedron	Rhombi-cuboctahedron	Truncated cuboctahedron	Snub cube	Tetrahedron	Icosahedron
Image
Coxeter diagram
Wythoff symbol	3 | 2 4	3 2 | 4	2 | 4 3	4 2 | 3	4 | 2 3	4 3 | 2	4 3 2 |	| 4 3 2
Symmetry group	Oh							O	Td	Th
	*432							432	*332	3*2
	[4,3]							[4,3]+	[1+,4,3] =[3,3]	[4,3+]

Cartan matrices and determinants

Every Coxeter diagram has a corresponding Cartan matrix. All Coxeter group Cartan matrices are symmetric because their root vectors are normalized. The Cartan matrix element a_i,j = a_j,i = −2cos(π / p) where p is the branch order between the pairs of mirrors.

Rank 2 Coxeter diagrams and their corresponding Cartan matrices are given here. The Cartan matrices for higher rank groups can be as simply constructed with the matrix element pairs corresponding between each pair of mirrors.

The determinant the Cartan matrix determines whether a group is finite (positive), affine (zero), or hyperbolic (negative). A hyperbolic group is compact if all subgroups are finite (i.e. have positive determinants).

The determinant by infinite families by rank are:

• det(A₁ⁿ=[2^n-1]) = 2ⁿ (Finite for all n)
• det(A_n=[3^n-1]) = n+1 (Finite for all n)
• det(BC_n=[4,3^n-2]) = 2 (Finite for all n)
• det(D_n=[3^n-3,1,1]) = 4 (Finite for all n)

Determinants in exceptional series:

• det(E_n=[3^n-3,2,1]) = 9-n (Finite for E₃(=A₂A₁), E₄(=A₄), E₅(=D₅), E₆, E₇ and E₈, affine at E₉ (${\tilde {E}}_{8}$), hyperbolic at E₁₀)
• det([3^n-4,3,1]) = 2(8-n) (Finite for n=4 to 7, affine (${\tilde {E}}_{7}$), and hyperbolic at n=8.)
• det([3^n-4,2,2]) = 3(7-n) (Finite for n=4 to 6, affine (${\tilde {E}}_{6}$), and hyperbolic at n=7.)
• det(F_n=[3,4,3^n-3]) = 5-n (Finite for F₃(=B₃) to F₄, affine at F₅ (${\tilde {F}}_{4}$), hyperbolic at F₆)
• det(G_n=[6,3^n-2]) = 3-n (Finite for G₂, affine at G₃ (${\tilde {G}}_{2}$), hyperbolic at G₄)

Rank 2 Coxeter group diagrams

Symmetry order p	Group name	Coxeter diagram	Cartan matrix
			$\left[{\begin{matrix}2&a_{12}\\a_{21}&2\end{matrix}}\right]$	Determinant (4-a21*a12)
Finite (Determinant>0)
2	I2(2) = A1xA1		$\left[{\begin{smallmatrix}2&0\\0&2\end{smallmatrix}}\right]$	4
3	I2(3) = A2		$\left[{\begin{smallmatrix}2&-1\\-1&2\end{smallmatrix}}\right]$	3
4	I2(4) = BC2		$\left[{\begin{smallmatrix}2&-{\sqrt {2}}\\-{\sqrt {2}}&2\end{smallmatrix}}\right]$	2
5	I2(5) = H2		$\left[{\begin{smallmatrix}2&-\phi \\-\phi &2\end{smallmatrix}}\right]$	$4\sin ^{2}(\pi /5)$ =$(5-{\sqrt {5}})/2$ ~1.38196601125
6	I2(6) = G2		$\left[{\begin{smallmatrix}2&-{\sqrt {3}}\\-{\sqrt {3}}&2\end{smallmatrix}}\right]$	1
8	I2(8)		$\left[{\begin{smallmatrix}2&-2\cos(\pi /8)\\-2\cos(\pi /8)&2\end{smallmatrix}}\right]$	$2-{\sqrt {2}}$ ~0.58578643763
10	I2(10)		$\left[{\begin{smallmatrix}2&-2\cos(\pi /10)\\-2\cos(\pi /10)&2\end{smallmatrix}}\right]$	$4\sin ^{2}(\pi /10)$ =$(3-{\sqrt {5}})/2$ ~0.38196601125
12	I2(12)		$\left[{\begin{smallmatrix}2&-2\cos(\pi /12)\\-2\cos(\pi /12)&2\end{smallmatrix}}\right]$	$2-{\sqrt {3}}$ ~0.26794919243
p	I2(p)		$\left[{\begin{smallmatrix}2&-2\cos(\pi /p)\\-2\cos(\pi /p)&2\end{smallmatrix}}\right]$	$4\sin ^{2}(\pi /p)$
Affine (Determinant=0)
∞	I2(∞) = ${\tilde {I}}_{1}$ = ${\tilde {A}}_{1}$		$\left[{\begin{smallmatrix}2&-2\\-2&2\end{smallmatrix}}\right]$	0

Finite Coxeter groups

See also polytope families for a table of end-node uniform polytopes associated with these groups.

• Three different symbols are given for the same groups – as a letter/number, as a bracketed set of numbers, and as the Coxeter diagram.
• The bifurcated D_n groups is half or alternated version of the regular C_n groups.
• The bifurcated D_n and E_n groups are also labeled by a superscript form [3^a,b,c] where a,b,c are the numbers of segments in each of the three branches.

Connected finite Dynkin graphs up to (ranks 1 to 9)

Rank	Simple Lie groups			Exceptional Lie groups
	${A}_{1+}$	${BC}_{2+}$	${D}_{2+}$	${E}_{3-8}$	${F}_{3-4}$	${G}_{2}$	${H}_{2-4}$	${I}_{2}(p)$
1	A1=[]
2	A2=[3]	BC2=[4]	D2=A1xA1			G2=[6]	H2=[6]	I2[p]
3	A3=[32]	BC3=[3,4]	D3=A3	E3=A2A1	F3=BC3		H3
4	A4=[33]	BC4=[32,4]	D4=[31,1,1]	E4=A4	F4		H4
5	A5=[34]	BC5=[33,4]	D5=[32,1,1]	E5=D5
6	A6=[35]	BC6=[34,4]	D6=[33,1,1]	E6=[32,2,1]
7	A7=[36]	BC7=[35,4]	D7=[34,1,1]	E7=[33,2,1]
8	A8=[37]	BC8=[36,4]	D8=[35,1,1]	E8=[34,2,1]
9	A9=[38]	BC9=[37,4]	D9=[36,1,1]
10+	..	..	..	..

Affine Coxeter groups

Families of convex uniform Euclidean tessellations are defined by the affine Coxeter groups. These groups are identical to the finite groups with the inclusion of one added node. In letter names they are given the same letter with a "~" above the letter. The index refers to the finite group, so the rank is the index plus 1. (Ernst Witt symbols for the affine groups are given as also)

1. ${\tilde {A}}_{n-1}$: diagrams of this type are cycles. (Also P_n)
2. ${\tilde {C}}_{n-1}$ is associated with the hypercube regular tessellation {4, 3, ...., 4} family. (Also R_n)
3. ${\tilde {B}}_{n-1}$ related to C by one removed mirror. (Also S_n)
4. ${\tilde {D}}_{n-1}$ related to C by two removed mirrors. (Also Q_n)
5. ${\tilde {E}}_{6}$, ${\tilde {E}}_{7}$, ${\tilde {E}}_{8}$. (Also T₇, T₈, T₉)
6. ${\tilde {F}}_{4}$ forms the {3,4,3,3} regular tessellation. (Also U₅)
7. ${\tilde {G}}_{2}$ forms 30-60-90 triangle fundamental domains. (Also V₃)
8. ${\tilde {I}}_{1}$ is two parallel mirrors. ( = ${\tilde {A}}_{1}$ = ${\tilde {C}}_{1}$) (Also W₂)

Affine Dynkin graphs up to (2 to 10 nodes)

Rank	${\tilde {A}}_{1+}$ (P2+)	${\tilde {B}}_{3+}$ (S4+)	${\tilde {C}}_{1+}$ (R2+)	${\tilde {D}}_{4+}$ (Q5+)	${\tilde {E}}_{n}$ (Tn+1) / ${\tilde {F}}_{4}$ (U5) / ${\tilde {G}}_{2}$ (V3)
2	${\tilde {A}}_{1}$=[∞]		${\tilde {C}}_{1}$=[∞]
3	${\tilde {A}}_{2}$=[3[3]]		${\tilde {C}}_{2}$=[4,4]		${\tilde {G}}_{2}$=[6,3]
4	${\tilde {A}}_{3}$=[3[4]]	${\tilde {B}}_{3}$=[4,31,1]	${\tilde {C}}_{3}$=[4,3,4]
5	${\tilde {A}}_{4}$=[3[5]]	${\tilde {B}}_{4}$=[4,3,31,1]	${\tilde {C}}_{4}$=[4,32,4]	${\tilde {D}}_{4}$=[31,1,1,1]	${\tilde {F}}_{4}$=[3,4,3,3]
6	${\tilde {A}}_{5}$=[3[6]]	${\tilde {B}}_{5}$=[4,32,31,1]	${\tilde {C}}_{5}$=[4,33,4]	${\tilde {D}}_{5}$=[31,1,3,31,1]
7	${\tilde {A}}_{6}$=[3[7]]	${\tilde {B}}_{6}$=[4,33,31,1]	${\tilde {C}}_{6}$=[4,34,4]	${\tilde {D}}_{6}$=[31,1,32,31,1]	${\tilde {E}}_{6}$=[32,2,2]
8	${\tilde {A}}_{7}$=[3[8]]	${\tilde {B}}_{7}$=[4,34,31,1]	${\tilde {C}}_{7}$=[4,35,4]	${\tilde {D}}_{7}$=[31,1,33,31,1]	${\tilde {E}}_{7}$=[33,3,1]
9	${\tilde {A}}_{8}$=[3[9]]	${\tilde {B}}_{8}$=[4,35,31,1]	${\tilde {C}}_{8}$=[4,36,4]	${\tilde {D}}_{8}$=[31,1,34,31,1]	${\tilde {E}}_{8}$=[35,2,1]
10	${\tilde {A}}_{9}$=[3[10]]	${\tilde {B}}_{9}$=[4,36,31,1]	${\tilde {C}}_{9}$=[4,37,4]	${\tilde {D}}_{9}$=[31,1,35,31,1]
11	...	...	...	...

Hyperbolic Coxeter groups

There are many infinite hyperbolic Coxeter groups. Hyperbolic groups are categorized as compact or not, with compact groups having bounded fundamental domains. Compact hyperbolic groups exist of rank 3 to 5, and noncompact groups exist up to rank 10.

Compact

Rank 3

There are infinitely many compact hyperbolic Coxeter groups of rank 3, including linear and triangle graphs. See notably the hyperbolic triangle groups.

Compact hyperbolic Coxeter groups

Linear	Cyclic
∞ [p,q], : 2(p+q)<pq ... ... ...	∞ [(p,q,r)], : p+q+r>9 ...

Ranks 4–5

The fundamental domain of either of the two bifurcating groups, [5,3^1,1] and [5,3,3^1,1], is double that of a corresponding linear group, [5,3,4] and [5,3,3,4] respectively. Letter names are given by Johnson as extended Witt symbols.

Compact hyperbolic Coxeter groups

Dimension Hd	Rank	Total count	Linear	Bifurcating	Cyclic
H3	4	9	3: ${\bar {BH}}_{3}$ = [4,3,5]: ${\bar {K}}_{3}$ = [5,3,5]: ${\bar {J}}_{3}$ = [3,5,3]:	${\bar {DH}}_{3}$ = [5,31,1]:	${\widehat {AB}}_{3}$ = [(3,3,3,4)]:   ${\widehat {AH}}_{3}$ = [(3,3,3,5)]:   ${\widehat {BB}}_{3}$ = [(3,4,3,4)]: ${\widehat {BH}}_{3}$ = [(3,4,3,5)]: ${\widehat {HH}}_{3}$ = [(3,5,3,5)]:
H4	5	5	3: ${\bar {H}}_{4}$ = [3,3,3,5]: ${\bar {BH}}_{4}$ = [4,3,3,5]: ${\bar {K}}_{4}$ = [5,3,3,5]:	${\bar {DH}}_{4}$ = [5,3,31,1]:	${\widehat {AF}}_{4}$ = [(3,3,3,3,4)]:

Noncompact

The highest noncompact hyperbolic Coxeter group is rank 10.

Rank 3

Noncompact Coxeter groups of rank 3 include linear and triangle graphs with one or more infinite order branches: (p and q = 3,4,5...)

Linear graphs	Cyclic graphs
[p,∞] [∞,∞]	[(p,q,∞)] [(p,∞,∞)] [(∞,∞,∞)]

Ranks 4–10

There are a total of 58 noncompact hyperbolic Coxeter groups from rank 4 through 10. All 58 are grouped below in five categories. Letter symbols are given by Johnson as Extended Witt symbols, using PQRSTWUV from the affine Witt symbols, and adding LMNOXYZ. These hyperbolic groups are given an overline, or a hat, for purely cyclic graphs. The bracket notation from Coxeter is a linearized representation of the Coxeter group.

Hyperbolic noncompact groups

Rank	Total count	Groups
4	23	${\widehat {BR}}_{3}$ = [(3,3,4,4)]: ${\widehat {CR}}_{3}$ = [(3,4,4,4)]: ${\widehat {RR}}_{3}$ = [4[4]]: ${\widehat {AV}}_{3}$ = [(3,3,3,6)]: ${\widehat {BV}}_{3}$ = [(3,4,3,6)]: ${\widehat {HV}}_{3}$ = [(3,5,3,6)]: ${\widehat {VV}}_{3}$ = [(3,6,3,6)]:	${\bar {P}}_{3}$ = [3,3[3]]: ${\bar {BP}}_{3}$ = [4,3[3]]: ${\bar {HP}}_{3}$ = [5,3[3]]: ${\bar {VP}}_{3}$ = [6,3[3]]: ${\bar {DV}}_{3}$ = [6,31,1]: ${\bar {O}}_{3}$ = [3,41,1]: ${\bar {M}}_{3}$ = [41,1,1]:	${\bar {R}}_{3}$ = [3,4,4]: ${\bar {N}}_{3}$ = [4,4,4]: ${\bar {V}}_{3}$ = [3,3,6]: ${\bar {BV}}_{3}$ = [4,3,6]: ${\bar {HV}}_{3}$ = [5,3,6]: ${\bar {Y}}_{3}$ = [3,6,3]: ${\bar {Z}}_{3}$ = [6,3,6]:	${\bar {DP}}_{3}$ = [3[ ]x[ ]]: ${\bar {PP}}_{3}$ = [3[3,3]]:
5	9	${\bar {P}}_{4}$ = [3,3[4]]: ${\bar {BP}}_{4}$ = [4,3[4]]: ${\widehat {FR}}_{4}$ = [(3,3,4,3,4)]: ${\bar {DP}}_{4}$ = [3[3]x[ ]]:	${\bar {N}}_{4}$ = [4,/3\,3,4]: ${\bar {O}}_{4}$ = [3,4,31,1]: ${\bar {S}}_{4}$ = [4,32,1]:	${\bar {R}}_{4}$ = [3,4,3,4]:	${\bar {M}}_{4}$ = [4,31,1,1]:
6	12	${\bar {P}}_{5}$ = [3,3[5]]: ${\widehat {AU}}_{5}$ = [(3,3,3,3,3,4)]: ${\widehat {AR}}_{5}$ = [(3,3,4,3,3,4)]:	${\bar {S}}_{5}$ = [4,3,32,1]: ${\bar {O}}_{5}$ = [3,4,31,1]: ${\bar {N}}_{5}$ = [4,3,/3\,3,4]:	${\bar {U}}_{5}$ = [3,3,3,4,3]: ${\bar {X}}_{5}$ = [3,3,4,3,3]: ${\bar {R}}_{5}$ = [3,4,3,3,4]:	${\bar {Q}}_{5}$ = [32,1,1,1]: ${\bar {M}}_{5}$ = [4,3,31,1,1]: ${\bar {L}}_{5}$ = [31,1,1,1,1]:
7	3	${\bar {P}}_{6}$ = [3,3[6]]:	${\bar {Q}}_{6}$ = [31,1,3,32,1]:	${\bar {S}}_{6}$ = [4,3,3,32,1]:
8	4	${\bar {P}}_{7}$ = [3,3[7]]:	${\bar {Q}}_{7}$ = [31,1,32,32,1]:	${\bar {S}}_{7}$ = [4,33,32,1]:	${\bar {T}}_{7}$ = [33,2,2]:
9	4	${\bar {P}}_{8}$ = [3,3[8]]:	${\bar {Q}}_{8}$ = [31,1,33,32,1]:	${\bar {S}}_{8}$ = [4,34,32,1]:	${\bar {T}}_{8}$ = [34,3,1]:
10	3		${\bar {Q}}_{9}$ = [31,1,34,32,1]:	${\bar {S}}_{9}$ = [4,35,32,1]:	${\bar {T}}_{9}$ = [36,2,1]:

Lorentzian groups

Coxeter groups can be defined as graphs beyond the hyperbolic forms. These can be considered to be related to a Lorentzian geometry, named after Hendrik Lorentz in the field of special and general relativity space-time, containing one (or more) time-like dimensional components whose self dot products are negative.

One usage includes the "very-extended" definitions which adds a third node to the over-extended simple groups. These extensions are usually marked by an exponent of 1,2, or 3 + symbols for the number of extended nodes. This extending series can be extended backwards, by sequentially removing the nodes from the same position in the graph, although the process stops after removing branching node. The E₈ extended family is the most commonly shown example extending backwards from E₃ and forwards to E₁₁.

The extending process can define a limited series of Coxeter graphs that progress from finite to affine to hyperbolic to Lorentzian. The determinant of the Cartan matrices determine where the series changes from finite (positive) to affine (zero) to hyperbolic (negative), and ending as a Lorentzian group, containing at least one hyperbolic subgroup. The noncrystalographic H_n groups forms an extended series where H₄ is extended as a compact hyperbolic and over-extended into a lorentzian group.

Smaller extended series

Finite	$A_{2}$	$C_{2}$	$G_{2}$	$A_{3}$	$B_{3}$	$C_{3}$	$H_{4}$
Rank n	[3[3],3n-3]	[4,4,3n-3]	Gn=[6,3n-2]	[3[4],3n-4]	[4,31,n-3]	[4,3,4,3n-4]	Hn=[5,3n-2]
2	[3] A2	[4] C2	[6] G2		[2] A12	[4] C2	[5] H2
3	[3[3]] A2+=${\tilde {A}}_{2}$	[4,4] C2+=${\tilde {C}}_{2}$	[6,3] G2+=${\tilde {G}}_{2}$	[3,3]=A3	[4,3] B3	[4,3] C3	[5,3] H3
4	[3[3],3] A2++=${\bar {P}}_{3}$	[4,4,3] C2++=${\bar {R}}_{3}$	[6,3,3] G2++=${\bar {V}}_{3}$	[3[4]] A3+=${\tilde {A}}_{3}$	[4,31,1] B3+=${\tilde {B}}_{3}$	[4,3,4] C3+=${\tilde {C}}_{3}$	[5,3,3] H4
5	[3[3],3,3] A2+++	[4,4,3,3] C2+++	[6,3,3,3] G2+++	[3[4],3] A3++=${\bar {P}}_{4}$	[4,32,1] B3++=${\bar {S}}_{4}$	[4,3,4,3] C3++=${\bar {R}}_{4}$	[5,33] H5=${\bar {H}}_{4}$
6				[3[4],3,3] A3+++	[4,33,1] B3+++	[4,3,4,3,3] C3+++	[5,34] H6
Det(Mn)	3(3-n)	2(3-n)	3-n	4(4-n)	2(4-n)

Middle extended series

Finite	$A_{4}$	$B_{4}$	$C_{4}$	$D_{4}$	$F_{4}$	$A_{5}$	$B_{5}$	$C_{5}$	$D_{5}$
Rank n	[3[5],3n-5]	[4,3,3n-4,1]	[4,3,3,4,3n-5]	[3n-4,1,1,1]	[3,4,3n-3]	[3[6],3n-6]	[4,3,3,3n-5,1]	[4,3,3,3,4,3n-6]	[31,1,3,3n-5,1]
3		[4,3-1,1] B2A1	[4,3] BC3	[3-1,1,1,1] A13	[3,4] BC3			[4,3,3] C3
4	[33] A4	[4,3,3] B4	[4,3,3] C4	[30,1,1,1] D4	[3,4,3] F4		[4,3,3,3-1,1] B3A1	[4,3,3,3] C4	[31,1,3,3-1,1] A3A1
5	[3[5]] A4+=${\tilde {A}}_{4}$	[4,3,31,1] B4+=${\tilde {B}}_{4}$	[4,3,3,4] C4+=${\tilde {C}}_{4}$	[31,1,1,1] D4+=${\tilde {D}}_{4}$	[3,4,3,3] F4+=${\tilde {F}}_{4}$	[34] A5	[4,3,3,3,3] B5	[4,3,3,3,3] C5	[31,1,3,3] D5
6	[3[5],3] A4++=${\bar {P}}_{5}$	[4,3,32,1] B4++=${\bar {S}}_{5}$	[4,3,3,4,3] C4++=${\bar {R}}_{5}$	[32,1,1,1] D4++=${\bar {Q}}_{5}$	[3,4,33] F4++=${\bar {U}}_{5}$	[3[6]] A5+=${\tilde {A}}_{5}$	[4,3,3,31,1] B5+=${\tilde {B}}_{5}$	[4,3,3,3,4] C5+=${\tilde {C}}_{5}$	[31,1,3,31,1] D5+=${\tilde {D}}_{5}$
7	[3[5],3,3] A4+++	[4,3,33,1] B4+++	[4,3,3,4,3,3] C4+++	[33,1,1,1] D4+++	[3,4,34] F4+++	[3[6],3] A5++=${\bar {P}}_{6}$	[4,3,3,32,1] B5++=${\bar {S}}_{6}$	[4,3,3,3,4,3] C5++	[31,1,3,32,1] D5++=${\bar {Q}}_{6}$
8						[3[6],3,3] A5+++	[4,3,3,33,1] B5+++		[31,1,3,33,1] D5+++
Det(Mn)	5(5-n)	2(5-n)		4(5-n)	5-n	6(6-n)	2(6-n)		4(6-n)

Some higher extended series

Finite	$A_{6}$	$B_{6}$	$D_{6}$	$E_{6}$	$A_{7}$	$B_{7}$	$D_{7}$	$E_{7}$	$E_{8}$
Rank n	[3[7],3n-7]	[4,33,3n-6,1]	[31,1,3,3,3n-6,1]	[3n-5,2,2]	[3[8],3n-8]	[4,34,3n-7,1]	[31,1,3,3,3,3n-7,1]	[3n-5,3,1]	En=[3n-4,2,1]
3									[3-1,2,1] E3=A2A1
4				[3-1,2,2] A22				[3-1,3,1] A3A1	[30,2,1] E4=A4
5		[4,3,3,3,3-1,1] B4A1	[31,1,3,3,3-1,1] D4A1	[30,2,2] A5				[30,3,1] A5	[31,2,1] E5=D5
6	[35] A6	[4,34] B6	[31,1,3,3,3] D6	[31,2,2] E6		[4,3,3,3,3,3-1,1] B5A1	[31,1,3,3,3,3-1,1] D5A1	[31,3,1] D6	[32,2,1] E6
7	[3[7]] A6+=${\tilde {A}}_{6}$	[4,33,31,1] B6+=${\tilde {B}}_{6}$	[31,1,3,3,31,1] D6+=${\tilde {D}}_{6}$	[32,2,2] E6+=${\tilde {E}}_{6}$	[36] A7	[4,35] B7	[31,1,3,3,3,30,1] D7	[32,3,1] E7	[33,2,1] E7
8	[3[7],3] A6++=${\bar {P}}_{7}$	[4,33,32,1] B6++=${\bar {S}}_{7}$	[31,1,3,3,32,1] D6++=${\bar {Q}}_{7}$	[33,2,2] E6++=${\bar {T}}_{7}$	[3[8]] A7+=${\tilde {A}}_{7}$	[4,34,31,1] B7+=${\tilde {B}}_{7}$	[31,1,3,3,3,31,1] D7+=${\tilde {D}}_{7}$	[33,3,1] E7+=${\tilde {E}}_{7}$	[34,2,1] E8
9	[3[7],3,3] A6+++	[4,33,33,1] B6+++	[31,1,3,3,33,1] D6+++	[34,2,2] E6+++	[3[8],3] A7++=${\bar {P}}_{8}$	[4,34,32,1] B7++=${\bar {S}}_{8}$	[31,1,3,3,3,32,1] D7++=${\bar {Q}}_{8}$	[34,3,1] E7++=${\bar {T}}_{8}$	[35,2,1] E9=E8+=${\tilde {E}}_{8}$
10					[3[8],3,3] A7+++	[4,34,33,1] B7+++	[31,1,3,3,3,33,1] D7+++	[35,3,1] E7+++	[36,2,1] E10=E8++=${\bar {T}}_{9}$
11									[37,2,1] E11=E8+++
Det(Mn)	7(7-n)	2(7-n)	4(7-n)	3(7-n)	8(8-n)	2(8-n)	4(8-n)	2(8-n)	9-n

Geometric folding

Finite and affine foldings

φA : AΓ --> AΓ' for finite types
Γ	Γ'	Folding description	Coxeter-Dynkin diagrams
I2(h)	Γ(h)	Dihedral folding
BCn	A2n	(I,sn)
	Dn+1, A2n-1	(A3,+/-ε)
F4	E6	(A3,±ε)
H4	E8	(A4,±ε)
H3	D6
H2	A4
G2	A5	(A5,±ε)
	D4	(D4,±ε)
φ: AΓ+ --> AΓ'+ for affine types
${\tilde {A}}_{n-1}$	${\tilde {A}}_{kn-1}$	Locally trivial
${\tilde {B}}_{n}$	${\tilde {D}}_{2n+1}$	(I,sn)
	${\tilde {D}}_{n+1}$, ${\tilde {D}}_{2n}$	(A3,±ε)
${\tilde {C}}_{n}$	${\tilde {B}}_{n+1}$, ${\tilde {C}}_{2n}$	(A3,±ε)
	${\tilde {C}}_{2n+1}$	(I,sn)
${\tilde {C}}_{n}$	${\tilde {A}}_{2n+1}$	(I,sn) & (I,s0)
	${\tilde {A}}_{2n}$	(A3,ε) & (I,s0)
	${\tilde {A}}_{2n-1}$	(A3,ε) & (A3,ε')
${\tilde {C}}_{n}$	${\tilde {D}}_{n+2}$	(A3,-ε) & (A3,-ε')
${\tilde {C}}_{2}$	${\tilde {D}}_{5}$	(I,s1)
${\tilde {F}}_{4}$	${\tilde {E}}_{6}$, ${\tilde {E}}_{7}$	(A3,±ε)
${\tilde {G}}_{2}$	${\tilde {D}}_{6}$, ${\tilde {E}}_{7}$	(A5,±ε)
	${\tilde {B}}_{3}$, ${\tilde {F}}_{4}$	(B3,±ε)
	${\tilde {D}}_{4}$, ${\tilde {E}}_{6}$	(D4,±ε)

Ver também: Dynkin diagram § Folding

A (simply-laced) Coxeter–Dynkin diagram (finite or affine) that has a symmetry (satisfying one condition, below) can be quotiented by the symmetry, yielding a new, generally multiply laced diagram, with the process called "folding".

For example, in D₄ folding to G₂, the edge in G₂ points from the class of the 3 outer nodes (valence 1), to the class of the central node (valence 3).

Geometrically this corresponds to orthogonal projections of uniform polytopes. Notably, any finite simply-laced Coxeter–Dynkin diagram can be folded to I₂(h), where h is the Coxeter number, which corresponds geometrically to a projection to the Coxeter plane.

A few hyperbolic foldings

See also

• Coxeter group
• Dynkin diagram
• Uniform polytope
  • Wythoff symbol
  • Uniform polyhedron
  • List of uniform polyhedra
  • List of uniform planar tilings
  • Uniform polychoron
  • Convex uniform honeycomb
  • Convex uniform honeycombs in hyperbolic space
• Wythoff construction and Wythoff symbol

References

• James E. Humphreys, Reflection Groups and Coxeter Groups, Cambridge studies in advanced mathematics, 29 (1990)
• Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1], Googlebooks [2]
  • (Paper 17) Coxeter, The Evolution of Coxeter-Dynkin diagrams, [Nieuw Archief voor Wiskunde 9 (1991) 233-248]
• Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999, ISBN 978-0-486-40919-1 (Chapter 3: Wythoff's Construction for Uniform Polytopes)
• Coxeter, Regular Polytopes (1963), Macmillian Company
  • Regular Polytopes, Third edition, (1973), Dover edition, ISBN 0-486-61480-8 (Chapter 5: The Kaleidoscope, and Section 11.3 Representation by graphs)
• Norman Johnson, Geometries and Transformations, Chapters 11,12,13, preprint 2011

External links

• Weisstein, Eric W. «Coxeter–Dynkin diagram» (em inglês). MathWorld 
• October 1978 discussion on the history of the Coxeter diagrams by Coxeter and Dynkin in Toronto, Canada; Eugene Dynkin Collection of Mathematics Interviews, Cornell University Library.